Syllabus and Notes for JSOM Ph.D. Math Refresher
This post provides the syllabus and is used to record my notes of JSOM Ph.D Math Refresher 2019.
For clear pdf version notes, you can download it here for you reference.
Course
Course Title: Math Refresher
Term: August 2019
Naveen Jindal School of Management, The University of Texas at Dallas
Version: June 11, 2019
Course Meeting Time & Location
Dates: August 5, 2018 to August 17, 2019
Location: JSOM 2.801
Contact Information
Instructor: Dr. Kyle Hyndman
Email: KyleB.Hyndman@utdallas.edu Office: JSOM 3.614
Instructor: Dr. Milind Dawande
Email: milind@utdallas.edu Office: JSOM 3.405
Course Description
This refresher course provides a refresher/introduction to the various mathematical tools that will be used in the core classes of the Ph.D. program (Management Science) at the Naveen Jindal School of Management. Topics include set theory, open and closed sets, sequences and limits, compactness, properties of functions (continuity, differentiability, (quasi-)concavity, metric spaces, linear algebra, etc. Emphasis will be placed on developing and using formal mathematical reasoning to prove results.
Student Learning Objectives
Apply formal mathematical reasoning to write logically consistent proofs of important mathematical results.
Refresh mathematical skills that will be used in MECO 6345 (Advanced Managerial Economics) and OPRE 7353 (Optimization) and throughout the Ph.D.
Textbook and Materials
There is no textbook for the refresher but we can provide some recommendations, particularly for those for which the content is new.
Ok, Efe A., Real Analysis with Economic Applications, Princeton University Press.
Simon, Carl P. and Lawrence Blume, Mathematics for Economists, Norton.
Strichartz, Robert S., The Way of Analysis, Jones and Bartlett.
For a much less advanced treatment, but one which should provide at least cursory coverage of all material, the following may be useful:
- Hoy, Michael, John Livernois, Chris McKenna, Ray Rees and Thanasis Stengos, Mathematics for Economics, Second Edition, MIT Press.
There may be more recent editions of each of these books. The exact edition you get is not important, nor is it required that you get any of these books.
Tentative Schedule
| Meeting | Date | Instructor | Topic |
|---|---|---|---|
| 1 | Aug 05 (M/A) | Daw | Intro. to Mathematical Reasoning, Set Theory, Sequences |
| 2 | Aug 06 (A, 2:30PM) | Daw | Problem Solving Session |
| 3 | Aug 07 (A) | Daw | Convex Sets |
| 4 | Aug 08 (M/A) | Hyn | Functions and Their Properties |
| 5 | Aug 9 (A) | Hyn | Problem Solving Session |
| 6 | Aug 10 (M/A) | Hyn | More on Functions; Metric Spaces and Compactness |
| 7 | Aug 12 (A) | Hyn | Problem Solving Session |
| 8a | Aug 13 (M) | Daw | Problem Solving Session |
| 8b | Aug 13 (A) | Hyn | Advanced Topics (Correspondences, Fixed Points) |
| 9 | Aug 14 (M/A) | Daw | Matrices, Vector Spaces, Linear Algebra |
| 10 | Aug 17 (A) | Hyn | Exam |
Notes: 1. “M” refers to a morning session, while “A” refers to an afternoon session. 2. There will be no classes on August 15 or 16, 2019 as there are mandatory PhD program and TA/RA orientations for those days.
Course Structure
Days with both a morning and afternoon session will primarily consist of more traditional lectures where topics are introduced and formal results are proven. Students are encouraged to participate fully in these sessions with questions as well as contributions for proving results. At the end of each full day, students will be given questions which will be discussed in the following afternoon session. Students should make a good faith effort to answer as many of the questions as possible before the problem solving session. During the problem solving session, all students should be prepared to offer solutions, advice and encouragement regarding the problems being discussed.
Student Deliverables
In the last session, there will be a short test that students must complete. This results of this test will be passed along to the respective Ph.D. Coordinators for each area. Furthermore, we stress that material from the bootcamp may return on the preliminary exam that JSOM students take at the end of the first year.
Lecture Notes




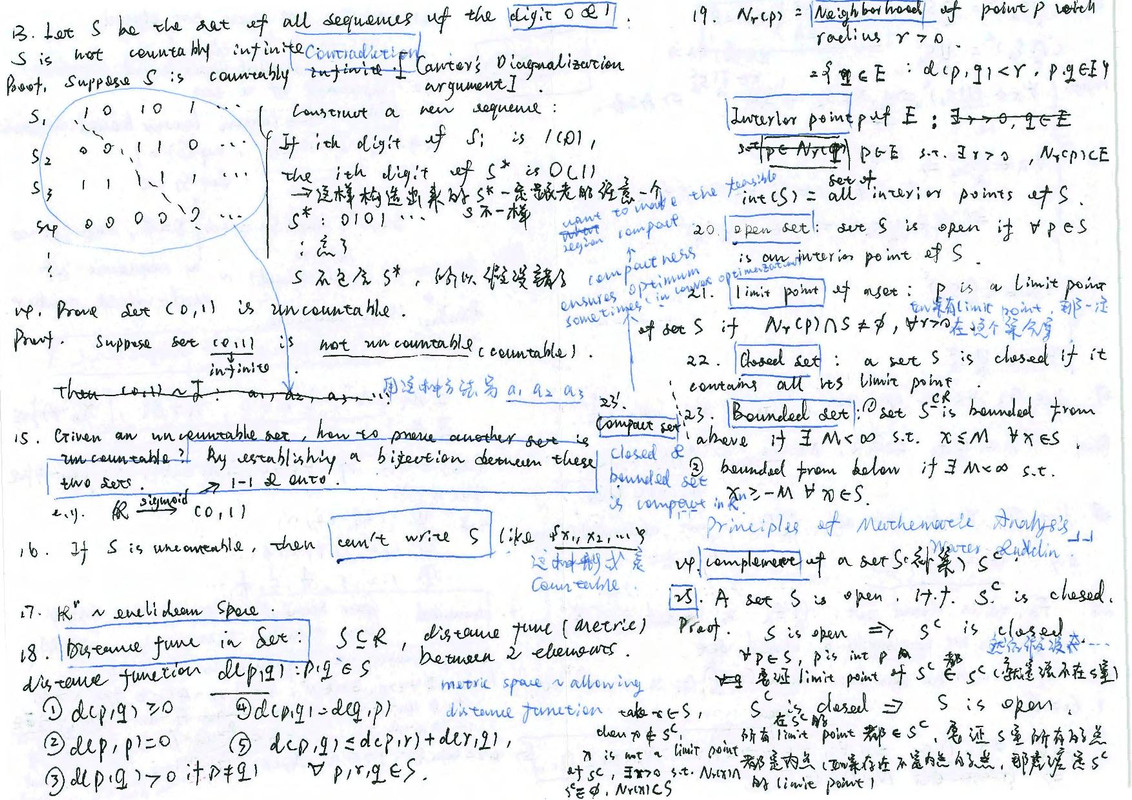















Syllabus and Notes for JSOM Ph.D. Math Refresher
https://blog.yihong-liu.com/2019/09/01/Syllabus-Notes-for-JSOM-Ph-D-Math-Refresher/
